Compressible Fluid Flow Equations
Here e is an internal energy per unit mass of fluid. They correspond to the Navier-Stokes equations with zero viscosity although they are usually written in the form shown here because this emphasizes the fact that they directly represent conservation of mass momentum and energy.
In fluid mechanics or more generally continuum mechanics incompressible flow isochoric flow refers to a flow in which the material density is constant within a fluid parcelan infinitesimal volume that moves with the flow velocityAn equivalent statement that implies incompressibility is that the divergence of the flow velocity is zero see the derivation below which illustrates why.

. Variables The units shown for the variables are SI International System of Units. Energy loss can be measured like static pressure drop in the direction of fluid flow with two gauges. If fully turbulent flow Re 10 8 and 0 εD 005 then Streeter et al.
Projects with complex fluid-flow systems. Eulers equations for compressible fluids written in Eulerian form. This book covers many basic and important concepts of fluid mechanics such as fluid statics potential flow compressible flows in one-dimensional and two-dimensional and multi-phase flow.
Incompressible flow reduces the continuity equation for conservation of mass to a divergenceless equation and this greatly simplifies the Navier-Stokes equations. The CFD Module provides rotating machinery interfaces that formulate the fluid flow equations in rotating frames available for both laminar and turbulent flow. Modern CFD software including grid generation and flow visualization tools will be used.
Some equations do not have equation number assigned. Provides FVM Matlab code involving implementation details of various numerics along with source code of the incompressible and compressible flow solvers developed in the book for OpenFOAM. The Continuum Hypothesis and Rarefied Flows.
Eulers equations in fluid dynamics describe the flow of a fluid without accounting for the fluids viscosity. The above set of equations is valid for compressible or incompressible inviscid flows. In physics and engineering fluid dynamics is a subdiscipline of fluid mechanics that describes the flow of fluidsliquids and gasesIt has several subdisciplines including aerodynamics the study of air and other gases in motion and hydrodynamics the study of liquids in motion.
An introduction to computational fluid dynamics CFD in mechanical engineering. Flow in pipe is always creating energy loss due to friction. General conditions are sought for the local and global conservation of primary mass and momentum and secondary kinetic energy.
Multiphase compressible high Mach number and thin film flows as well as the shallow water equations the CFD Module provides a large number of fluid flow interfaces tailored for. They are adequate for an entry-level course. Fluid dynamics has a wide range of applications including calculating forces and moments on.
Mass flow rate density at standard conditions and flow rate at standard conditions are. In fluid dynamics the Euler equations govern the motion of a compressible inviscid fluid. The theory and numerical techniques of CFD.
However the equations above are valid for any consistent set of units. The spatial discretization of convective terms in compressible flow equations is studied from an abstract viewpoint for finite-difference methods and finite-volume type formulations with cell-centered numerical fluxes. General equation for pressure drop known as Darcys formula expressed in meters of fluid is.
The coupled system of equations is discretized in time by use of an implicit Euler scheme CD-Adapco 2019A small physical time step of Δ t ˆ 1 1 0 6 s has been selected to capture high-frequency turbulent behavior of the flow which is comparable to that used in relevant valve literature Yonezawa et al 2010a Wang and Liu 2017 Wang et al 2019. Fluid dynamics are often differentiated into compressible and incompressible flows each of which may be viscous or inviscid. The flow equations Equation rely on the continuum hypothesis that is a fluid can be regarded as a continuum rather than a collection of individual moleculesFlows where molecular effects are of significance are known as rarefied flowsThe degree of rarefaction is measured by the Knudsen number.
2 The unit in some tables. Detailed treatment of SIMPLE-based all speed flow algorithms and role of the Rhie-Chow interpolation.
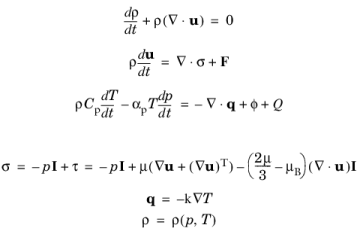
General Linearized Compressible Flow Equations

Bernoulli S Equation For Compressible Fluid Flow Understanding Business Analysis And Engineering Principles
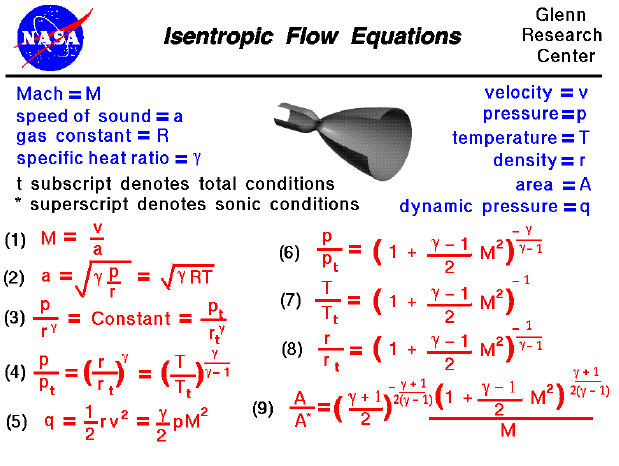
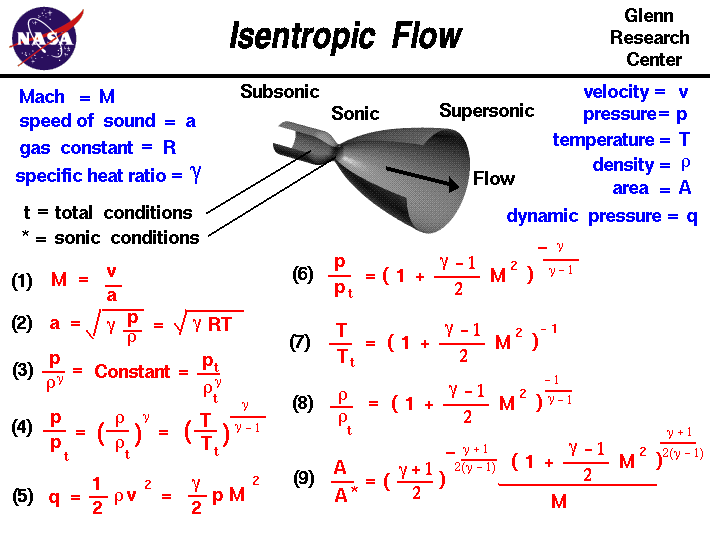
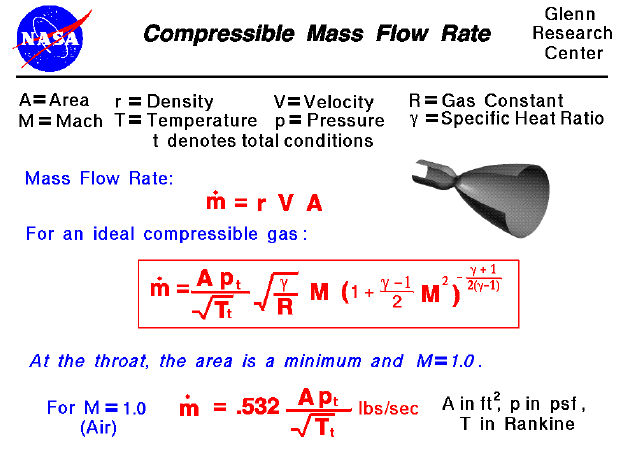
0 Response to "Compressible Fluid Flow Equations"
Post a Comment